Dear all,
I apologize for the long text.
I’m working with S1GRD products. I’m trying to understand:
-
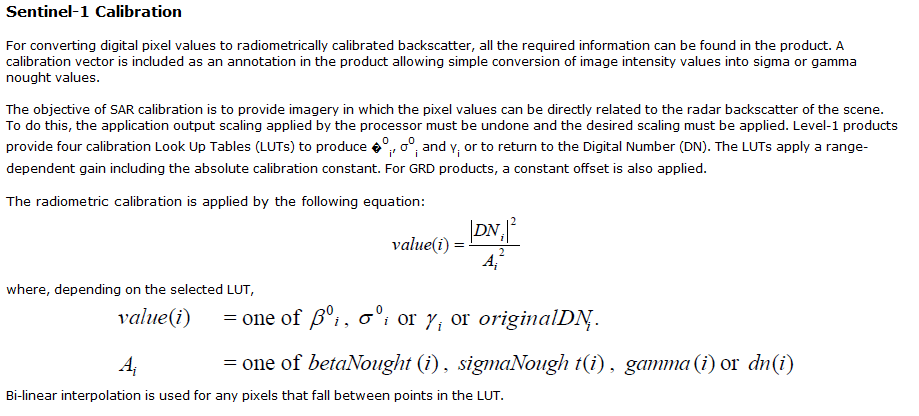
how the calibration works in general
-
the difference between Beta0, Sigma0, Gamma0
-
if the Local Incidence Angle is really taken in account for the calculation of Sigma0 (and Gamma0) and which are the effects of this correction.
In order to obtain and analyze the Calibration outputs (Beta0, Sigma0, Gamma0) I’ve prepared the following (simple) workflow:
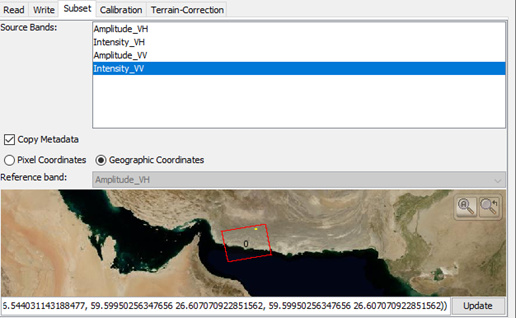
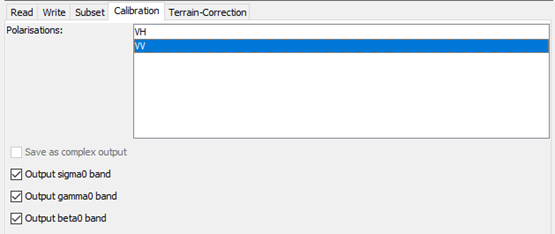

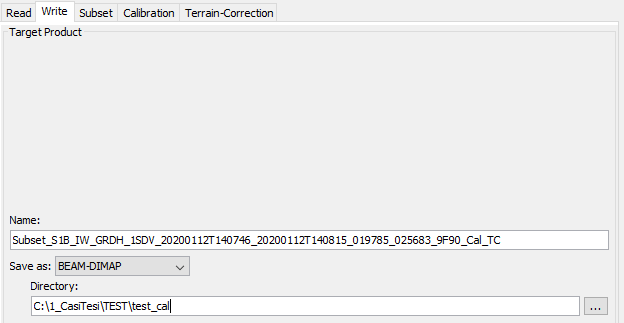
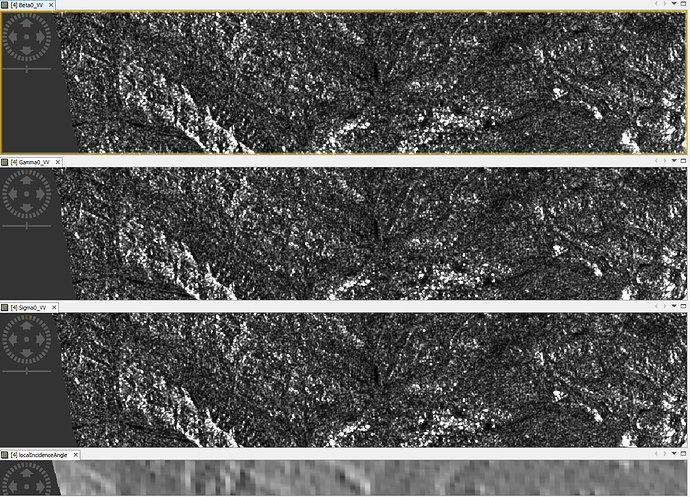
By a first general visual analysis of the outputs is possible to note that Beta0, Sigma0 and Gamma0 are more or less identical (Beta0 is slightly darker than Sigma0 and Gamma0).
Below also the calculated statistics for each “band”:
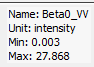
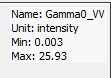
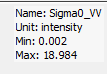
The output similarity surprised me considering that the outputs are obtained by applying 3 different formula.
My first question:
1- The results of these outputs, is it plausible?
Let’s focus now on Sigma0.
I’d like to understand if the Local Incidence Angle is taken into account during the Calibration in Sigma0.
For my purpose, I need the Sigma0 formula and a definition.
Regarding the formula, searching through the Forum and by consulting technical radar documentation, I’ve found two versions:
First version
![]()
Sources:
Second version
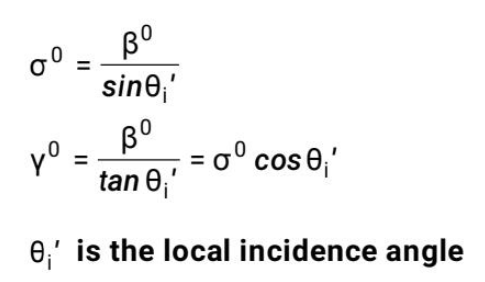
Sources:
Also consulting some Sigma0 definition, looks like the Local Incidence Angle is taken surely in account:
Thus, in order to ensure that local incidence angle has been considered during the calculation, I’m going “calculate by hand” Sigma0 for one pixel starting from Beta0 and using the value of the Local Indicence Angle obtained as output (hoping on its quality reliability):
I take into account the first formula (the most “popular”):
sigma0 = beta0 * sin(inc angle)
My sample values are the following:
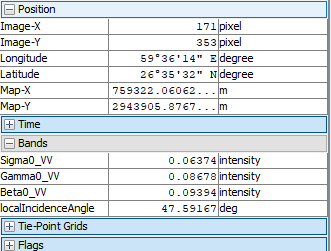
Thus, my expectation is to calculate a sigma0 value=0.06374
Applying the formula:
Sigma0= 0.09394 x sin(47.59) = 0.0693
The result is close enough to my expectation.
My second question:
2- Is the second formula [Sigma0=Beta0/sin(inc ang)] simply an Airbus mistake?
My last questions/considerations:
3-Can we consider Beta0 calibration as the basic one, obtained applied just a calibration constant?
4-Can we consider Sigma0 calibration as an improvement of Beta0, because the effects of the Local Incidence Angle are reduced (but not totally removed)?
5-Can we consider Gamma0 calibration as an improvement of Sigma0 because the effects of the Local Incidence Angle are well corrected than in Sigma0 (but not totally removed)?
*thx to @ABraun for the sources!
BR